
A thought experiment that’s dividing mathematicians can help illuminate how belief shapes rational decisions.
Usually, there are clear answers in mathematics—especially if the tasks are not too complicated. But when it comes to the Sleeping Beauty problem, which became popular in 2000, there is still no universal consensus.
Experts in philosophy and mathematics split into two camps and ceaselessly cite—often quite convincingly—arguments for their respective side. More than 100 technical publications exist on this puzzle, and almost every person who hears about the Sleeping Beauty thought experiment develops their own strong opinion.
The problem vexing the minds of experts is as follows: Sleeping Beauty agrees to participate in an experiment. On Sunday she is given a sleeping pill and falls asleep. One of the experimenters then tosses a coin. If “heads” comes up, the scientists awaken Sleeping Beauty on Monday.
Afterward, they administer another sleeping pill. If “tails” comes up, they wake Sleeping Beauty up on Monday, put her back to sleep and wake her up again on Tuesday. Then they give her another sleeping pill. In both cases, they wake her up again on Wednesday, and the experiment ends.
The important thing here is that because of the sleeping drug, Sleeping Beauty has no memory of whether she was woken up before. So when she wakes up, she cannot distinguish whether it is Monday or Tuesday. The experimenters do not tell Sleeping Beauty either the outcome of the coin toss nor the day.
They ask her one question after each time she awakens, however: What is the probability that the coin shows heads?
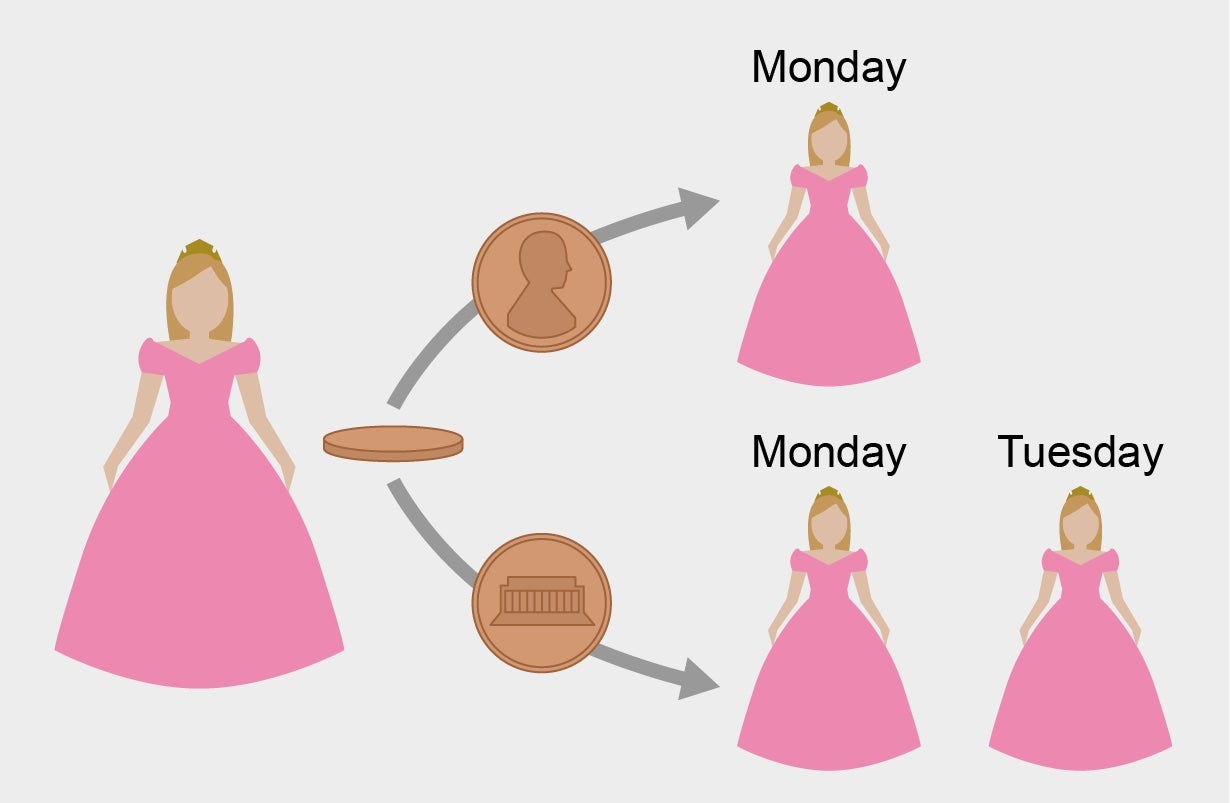
Put yourself in the position of Sleeping Beauty: You wake up, you don’t know what day it is, and you don’t know if you have been woken up before. You only know the theoretical course of the experiment.
My first intuition was that Sleeping Beauty should guess ½.The probability of the coin landing on heads or tails—regardless of the rest of the experiment—is always 50 percent. U.S. philosopher David Lewis held the same view when he learned of the problem.
After all, one could even flip the coin before sending Sleeping Beauty to sleep. By the experiment’s design, she does not have any extra clues to the situation, so logically she should state the probability as ½.
But there are also conclusive arguments in favor of a probability of ⅓. If you think through Sleeping Beauty’s experience, then three scenarios can occur:
She wakes up Monday, and heads was thrown.
She wakes up Monday, and tails was thrown.
She wakes up Tuesday, and tails was thrown.
What are the probabilities for each event? You can investigate this both mathematically and empirically. Suppose you flip a coin 100 times and get tails 52 times and heads 48 times. Put another way, the Monday/heads scenario occurs 48 times, and Monday/tails and Tuesday/tails occur 52 times each.
Because Tuesday/tails always follows Monday/tails, the probabilities for all three events are equal—and must therefore be ⅓. When Sleeping Beauty is awakened and asked to answer what the probability of the coin toss was for heads, she should therefore answer ⅓, according to this reasoning.


Philosopher of science Adam Elga of Princeton University, who popularized the Sleeping Beauty problem in 2000, came to this conclusion. He formulated his argument in a mathematically sound way.
If Sleeping Beauty is told when she wakes up that today is Monday (M), then the probability of Monday/heads (M, H) and Monday/tails (M, Z) is indisputably equal: P(M, H) = P(M, Z) = ½, where P stands for probability. On the other hand, if Sleeping Beauty wakes up and learns that tails have been thrown, then that day could equally be either Monday or Tuesday (T), meaning P(M, Z) = P(T, Z) = ½.
According to the calculus of conditional probabilities, it follows that in the general case (without Sleeping Beauty receiving any additional information), the three values are equal: P(M, Z) = P(M, H) = P(T, Z). Because all three probabilities must add up to 1, each individual value is ⅓. In other words, because Sleeping Beauty is awakened twice as often in the case of tails as in the case of heads, she should answer with ⅓, from Elga’s point of view.
Mathematicians taking it to the extreme
How would you answer the question now that you have heard the two main arguments? To get an even better sense of the Sleeping Beauty problem, it can help to think of a more extreme version of the thought experiment.
Suppose that in the case of tails, Sleeping Beauty will be awakened and questioned not just one additional time the next day but a million times (presumably at smaller intervals—because, even for a fairy tale character, this schedule would be brutal).
If you wake her up and ask her the probability that the coin landed on heads, the answer ½ doesn’t seem logical in this scenario. If the coin toss results in tails, Sleeping Beauty is questioned a million times in a row, and in the case of heads, she is questioned just once.
But extreme cases can also strengthen the ½ camp’s position. For example, instead of a coin toss, a sports bet could be used, such as a footrace pitting retired sprinter Usain Bolt against singer Taylor Swift. In this scenario, if Bolt, a world record holder in multiple running categories, defeats the pop star—as most people would anticipate—Sleeping Beauty will only be awakened once on Monday.
But if, contrary to all expectations, Swift proves swifter, Sleeping Beauty must wake up every day for a month, 30 times in a row. The probability of Bolt losing to Swift is very low. But if we apply the same logic that motivated the ⅓ response, we need to treat those scenarios with equal weight. Sleeping Beauty would still have to bet on a Swift victory after waking up because in this—admittedly unlikely—situation, she could be awakened 30 times. Lewis found this argument nonsensical. This thought experiment, he thus contended, supports the ½ faction.
Are you now completely confused? You are not alone. Has your opinion changed? Mine has. I’m no longer completely convinced by the ½ camp, at any rate. I can also gain insight from the ⅓ position.
This puzzle has some interesting applications. Philosophers and mathematicians can use it to think about decision-making and probability broadly. For example, this thought experiment illustrates how someone’s beliefs—in this case, Sleeping Beauty’s—can lead to more than one rational conclusion. It also underscores the difference between the number of experimental possibilities (such as flipping heads versus tails) and the possible experiences of someone within an experiment.
SOURCE: SCIENTIFIC AMERICAN
